
Now that we have found the value of 𝑏, we know the 𝑛 th term = 2 𝑛 2 + 1 For example, the sequence 2, 4, 6, 8, 10 is an arithmetic sequence because the numbers increase by a fixed amount (2) each time. We look at the pupils progression like an arithmetic sequence and aim to build in gradual learning section-by-section in order to achieve a significant improvement by the end of the study task.The number sequences worksheet has been written for GCSE Foundation Maths learners - ages 14-16 - but can also be tackled by advanced KS3 Maths learners. A sequence is a set of numbers that follow a specific pattern or rule. In fact, whenever the second difference is a constant value it will be a quadratic sequence.
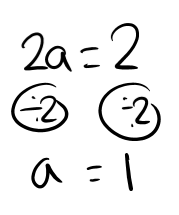
Quadratic sequences worksheet ks3 how to#
So, substituting that into the formula for the 𝑛 th term will help us to find the value of 𝑏: Sequences are a fundamental concept in mathematics, and understanding how to work with them is essential for success in KS3 and KS4 maths. This means that it is a quadratic sequence. We know that the 𝑛 th term = 2 𝑛 2 + 𝑏 𝑛 + 1 Section 2 contains 3 applied questions with a mix of worded problems and deeper problem solving. Section 1 of the quadratic sequences worksheet contains 27 skills-based questions, in 3 groups to support differentiation.
Quadratic sequences worksheet ks3 free#
So far… in the sequence: 3, 9, 19, 33, 51, … Help your students prepare for their Maths GCSE with this free quadratic sequences worksheet of 35 questions and answers.

Where 𝑎 is the 2 nd difference ÷ 2 and 𝑐 is the zeroth term We calculated the zeroth term as 1 and the 2 nd difference as 4. So the first difference between the terms in position 0 and 1 will be 6 − 4 = 2. Working backwards, we know the second difference will be 4. Unlike a linear sequence, the terms in a quadratic sequence do not have a common difference. Whether you want a homework, some cover work, or a lovely bit of extra practise, this is the place for you. A quadratic sequence is a sequence where the n th term rule includes an n 2 (remember, a term is the word for a number in a sequence). The zeroth term is the term which would go before the first term if we followed the pattern back. Quadratic Sequences: Worksheets with Answers. How do you find the 𝑛 th term of a quadratic sequence? We see why it’s called a quadratic sequence the 𝑛 th term has an 𝑛 2 in it. The 𝑛 th term of a quadratic sequence takes the form of: 𝑎 𝑛 2 + 𝑏 𝑛 + 𝑐. Variety of resources Ive made to teach all aspects of sequences, from term-to-term rule, to position-to-term rule, to nth terms of linear and quadratic sequences. What is the 𝑛 th term of a quadratic sequence? The terms of the sequence will alternate between positive and negative.Higher Sequences Digital Revision Bundle What is a quadratic sequence?Ī quadratic sequence is one whose first difference varies but whose second difference is constant. Generating Linear Sequences KS3 Walkthrough Worksheet. This pack includes a starter, teaching PowerPoint, lesson plan, worksheets and a handy how-to guide. Some of the terms of this sequence are surds, so leave your answer in surds as this is more accurate than writing them in decimal form as they would have to be rounded. An adaptable lesson pack, designed for experienced teachers, to support the teaching of finding the nth term of a quadratic sequence. Show that the sequence 3, 6, 12, 24, … is a geometric sequence, and find the next three terms.ĭividing each term by the previous term gives the same value: \(\frac\). Part 2: Finding the position to term rule of a quadratic sequence.

Four sequences, A, B, C and D, are defined by the following formulae: A. (d) Calculate the 58th term of the sequence defined by unn1000 5. (c) Calculate the 200th term of the sequence given by unn+322. (b) Calculate the 25th term of the sequence given by unn11 3. WALT and WILF Part 1: Using position to term rule to find the first few terms of a quadratic sequence. (a) Calculate the 100th term of the sequence given by unn85. In a \(geometric\) sequence, the term to term rule is to multiply or divide by the same value. Examples include the bar model to help pupils understand that the original amount equals 100 Worksheet Free MathsbyFintan.


 0 kommentar(er)
0 kommentar(er)
